
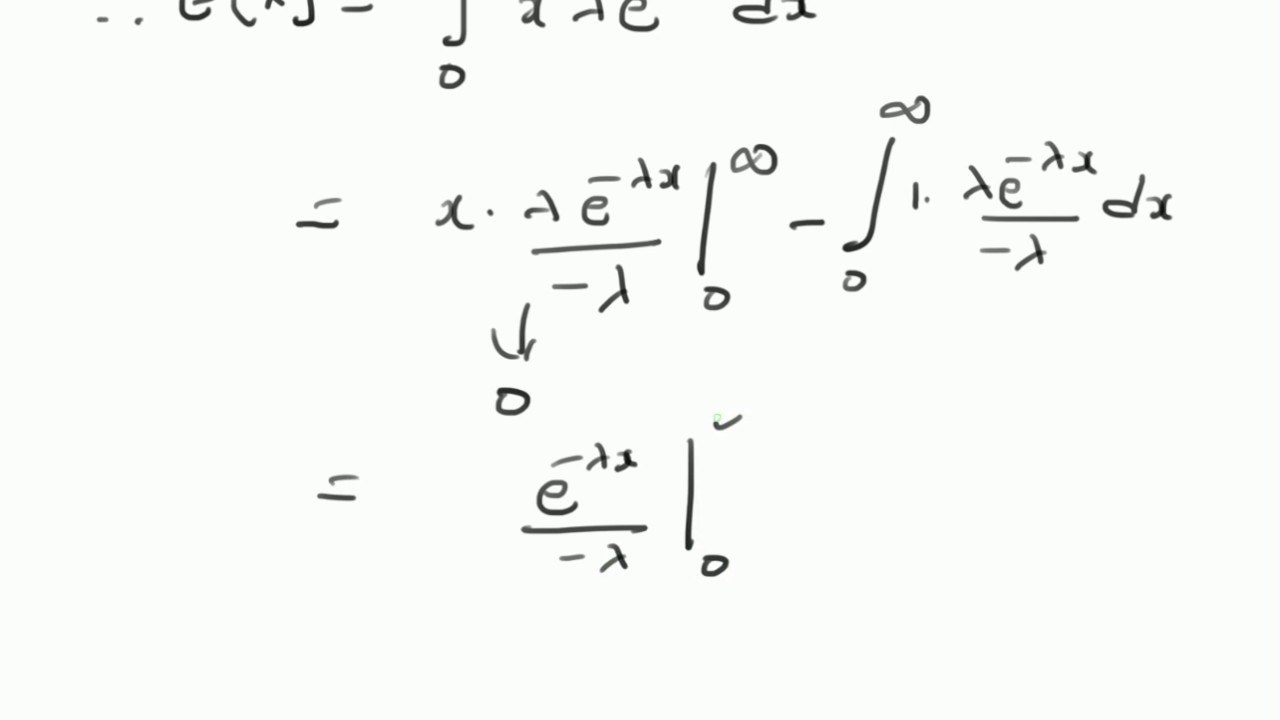
We see from Figure 2, that the fit for the data is a Weibull distribution with parameters α = 691.0264 and β = 3.7683. After pressing the OK button, the output shown in Figure 2 appears.įigure 2 – Fit for a Weibull distribution Definition of Generalized Method of Moments (GMM): An econometric method for estimating parameters in statistical models where the maximum likelihood. Chapter 1 INTRODUCTION TO THE GENERALIZED METHOD OF MOMENTS ESTIMATION David Harris and Lszl Mtys One of the most important tasks in econometrics and. This is implemented in Excel via the formulaĪs described in Weibull Distribution, we call Goal Seek by selecting Data > What If Analysis|Goal Seek and then filling in the dialog box that appears in Figure 1. We can now use Excel’s Goal Seek capability to find β. We initially set the value of the β parameter in cell H4 to some guess, i.e. You will learn about desirable properties that can be used to help you to differentiate between good and bad estimators. Cell E6 contains the formula on the left side of the equation that we derived above to find the β parameter, namely =GAMMALN(1+2/H4)-2*GAMMALN(1+1/H4)-LN(E3^2+E4^2)+2*LN(E3). In particular, cells E3 and E4 contain the formulas =AVERAGE(B4:B15) and STDEV.S(B4:B15). We implement these equations in Excel as shown in Figure 1. Using algebra, we can now eliminate α to obtain As we saw in Weibull Distribution, once we do this, we can estimate the scale and shape parameters based on the fact thatĮstimating μ by x̄ and σ by s, it then follows that We can estimate the mean μ and standard deviation σ of the population from the data in Figure 1. Find the scale and shape parameters that best fit the data.įigure 1 – Fitting a Weibull distribution Then a sample is drawn and the population moments are estimated from the. Gallant and Tauchen (1996a, 2002a) developed a systematic approach to generating moment conditions for the generalized method of moments (GMM) estimator (Hansen. One starts with deriving equations that relate the population moments (i.e., the expected values of powers of the random variable under consideration) to the parameters of interest. We believe that the data fits a Weibull distribution. In statistics, the method of moments is a method of estimation of population parameters. Generalized Moethod of Moments is a broadly applicable parameter estimation strategy which nests the classic method of moments, linear regression, max. The time to failure is shown in range B4:B15 of Figure 1. Elsewhere, we show two other approaches using the maximum likelihood method and regression.Įxample 1: Twelve robots were operated until they failed. We illustrate the method of moments approach on this webpage. A parametric model is a family of probability distributions that can be.
METHOD OF MOMENTS HOW TO
We will now turn to the question of how to estimate the parameter(s) of this distribution. Given a collection of data that may fit the Weibull distribution, we would like to estimate the parameters which best fit the data. Lecture 12 Parametric models and method of moments In the last unit, we discussed hypothesis testing, the problem of answering a binary question about the data distribution.


 0 kommentar(er)
0 kommentar(er)
